Welcome back to our series on GMAT circles. In the first article, we introduced the properties of radius/diameter, circumference, and area, discussing the relationships between all of these. This time we will introduce something called a central angle, which creates portions of a circle’s area and circumference called sectors and arcs, respectively.
The best way to define these things is probably with a simple visual.
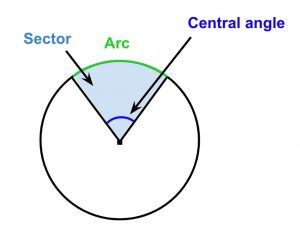
A central angle is an angle created by using line segments to connect a circle’s center to two points on its edge. A sector is the part of a circle’s area bounded by this central angle, and an arc is the part of a circle’s circumference between the two points used to draw the angle. A 90-degree central angle creates both a 90-degree sector and a 90-degree arc. An important note is that the lines used to form the central angle are radii of the circle.
As a further illustration, think about a pizza (something I do regularly). The pizza is a circle, the pieces are sectors separated by central angles, the crust is the circle’s circumference, and each piece’s section of crust is an arc. From this, you can see that any central angle creates both a sector and an arc that correspond to one another. When you pull a piece from a pizza or cut out a piece from a pie, you use a central angle to create a sector with an arc on its rounded edge.
To represent these things mathematically, we consider a circle to be like a 360-degree central angle. In this setup, the fractional relationship of a central angle to 360 corresponds to two things:
- The fractional relationship of the resulting sector to the circle’s total area
- The fractional relationship of the resulting arc to the circle’s total circumference
Since 90 is ¼ of 360, the area of a 90-degree sector is ¼ of its circle’s total area, and the length of a 90-degree arc is ¼ of its circle’s circumference.
To show all of this algebraically, let’s use the variable x for the degree measure of a central angle:
x / 360 = arc length / circumference
x / 360 = sector area / circle area
Most pizzas are divided into 8 slices. This means that each slice has a central angle of 360/8 = 45° and that each slice is ⅛ of the area of the entire pizza.
Examples:
1. What is the central angle for three slices of pizza?
The central angle formed by 3 slices of pizza is 3 * 360 / 8 = 135 degrees.
2. What’s the area of a slice if the diameter is 20cm and there are six slices?
The area of a slice of pizza is 1/6 of its pizza’s total area. So, the area of a pizza can be found by using this formula A= π*r2 = 3.14*102 = 314cm
The area of a slice of pizza is 314/ 6 = 52.33cm
Keep in mind that you may have to consider this relationship in either direction. You may be given some info about the whole circle and then tasked with concluding something about a sector or an arc. Or you may be given some info about a sector or an arc and then tasked with concluding something about the whole circle. You may even be given info about both the whole circle (its area or circumference) and a sector or arc and then tasked with calculating the central angle. Each of these represents a perspective shift, and when doing a problem form, you can rewrite the problem from each of these perspectives to make sure you can fully navigate problems of this sort.
Pieces of Pi: Official GMAT Problems
Now for some official GMAT problems. Let’s start with two straightforward sector problems, one problem solving and one data sufficiency.
Problem-Solving Problem
The annual budget of a certain college is to be shown on a circle graph. If the size of each sector of the graph is to be proportional to the amount of the budget it represents, how many degrees of the circle should be used to represent an item that is 15 percent of the budget?
A. 15°
B. 36°
C. 54°
D. 90°
E. 150°
From the question, we can tell that the “circle graph” mentioned here is what we usually call a “pie chart,” a handy way to show the breakdown of a whole (like a budget) into its various parts. If we want to represent 15% of the budget, we need a sector with a central angle using 15% of the (360) available degrees in the circle. 0.15 * 360 = 54, so the correct answer is C. Piece of cake. Or piece of pie.
Now for a DS pie chart problem
TOTAL EXPENSES FOR THE FIVE DIVERSIONS OF COMPANY H
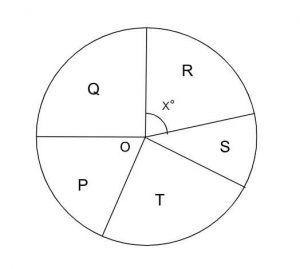 The figure represents a circle graph of Company’s H total expenses broken down by the expenses for each of its five diversions. If O is the center of the circle and if Company H’s total expenses are $5,400,000, what are the expenses for Division R?
The figure represents a circle graph of Company’s H total expenses broken down by the expenses for each of its five diversions. If O is the center of the circle and if Company H’s total expenses are $5,400,000, what are the expenses for Division R?
1. x = 94
2. The total expenses for Division S and T are twice as much as the expenses for Division R.
Once again, this pie chart (which the GMAT apparently prefers to call a “circle graph”) is being used to represent a budget breakdown. Here we are told that the value represented by the whole circle is $5,400,000. We can think of this value as the area of the circle. We are asked for the expenses for division R, or in circle terms, the area of sector R.
Statement 1: x = 94
This is the measure of the central angle bounding the sector whose area we need to know (sector R). Since we already know the area of the whole circle, the measure of this central angle is the final piece of the puzzle. (Area of sector R = 94/360 * $5,400,000) Statement 1 is sufficient.
Statement 2: The total expenses for Divisions S and T are twice as much as the expenses for Division R.
This statement relates the total of two unknown sectors to another unknown sector. Given this statement alone, we don’t know the relationship of any of these sectors to the whole circle, so we can’t solve for any of their areas. Statement 2 is insufficient.
The answer:
Statement 1 is sufficient.
Statement 2 is insufficient.
The correct answer is A.
Pieces of Pi: More Difficult Problems
Let’s ratchet up the difficulty a bit with another sector problem that involves more smoke and mirrors.
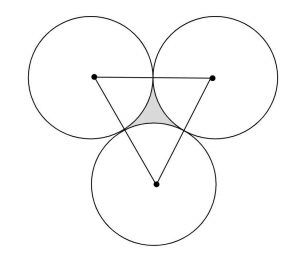
The figure consists of three identical circles that are tangent to each other. If the area of the shaded region is 64√3 – 32*π, what’s the radius of each circle?
A. 4
B. 8
C. 16
D. 24
E. 32
(tangent means just touching and not overlapping)
The problem mentions only three circles and a shaded region, but the graphic includes something more: an equilateral triangle drawn by connecting the centers of the three circles. You can solve this problem without knowing anything about the formula for the area of an equilateral triangle (although you should know this formula). It should occur to you that the area of the shaded region could be expressed as the area of the triangle minus the combined area of those three sectors, which matches the given expression 64√3 – 32*π. So the (irrelevant) area of the triangle is 64√3, and the area of the three sectors is 32*π.
You might start by trying to get the area of a single sector by dividing 32*π by 3. But 32 won’t divide nicely by 3, which should signal you to try something else. If you can’t go from the combined area of the three sectors to the area of one sector, maybe you can go from the combined area of the three sectors to the area of one circle. You might use your spatial reasoning and conclude that the three sectors together look like they make up half a circle. Or you might recall that each interior angle of an equilateral triangle measures 60 degrees. Therefore each of these sectors is ⅙ (60/360) of a whole circle, and the three of them together do indeed make up half a circle (3 * ⅙ = ½).
Now, if the sectors’ combined area is 32*π, and this is half a circle, then the area of the whole circle is 2 * 32*π = 64. Having found the area of a circle, we can now solve for the radius.
A = 64 = π*r2
64 = r2
r = 8
And the correct answer choice is B.
Arc Length Problem
Let’s try one more problem, this time focusing on arc length.
The points R, T, and U lie on a circle that has radius 4. If the length of arc RTU is 4*π/3 what is the length of line segment RU?
A. 4/3
B. 8/3
C. 3
D. 4
E. 6
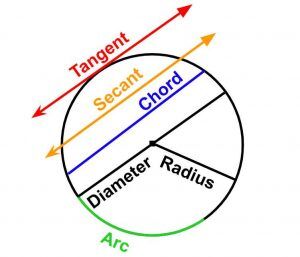
A note about points that “lie on a circle.” This always means that the points are on the edge or perimeter of the circle.
It may be helpful to visualize or even draw out what has been described here.
This is a good opportunity to introduce some terminology. We see that line segment RU connects two points on the circle. Such a line segment is called a chord. If the line continues on to either side of the circle so that the circle is “skewered,” the line is called a secant (the GMAT does not expect you to know this term). When a chord or secant passes through the circle’s center, it creates a diameter. A line outside a circle that just touches the circle at one point is called a tangent.
If you aren’t sure how to calculate the length of a chord like RU, start with what you know. We are given the length of arc RTU (4*π/3) and the radius of the circle. A good step is to calculate the circumference of the circle so that we can see how it relates to arc RTU.
C = 2*π*r
C = 2*π*4
C = 8*π
The circumference is 8*π, and arc RTU is 4*π/3. 8*π/6 = 4*π/3. Therefore arc RTU represents ⅙ of the circumference of the circle, and its corresponding central angle is 60 degrees (360/6). Drawing out this information helps us to see its relevance.
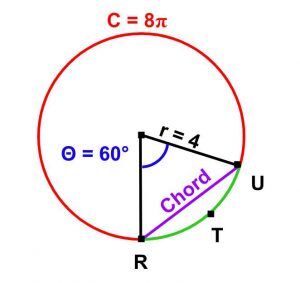 The central angle and chord RU form an equilateral triangle. Since the radius of the circle is 4, chord RU also has length 4, and the correct answer is D.
The central angle and chord RU form an equilateral triangle. Since the radius of the circle is 4, chord RU also has length 4, and the correct answer is D.
This concludes our second article on the GMAT’s treatment of circles. Next time we will look at another kind of angle inside a circle: an inscribed angle, and at the related topic of inscribed polygons.
Contributor: Elijah Mize (Apex GMAT Instructor)
