As discussed before, now that we’ve talked about the basic triangles, we can start looking at how the GMAT can make problems difficult by embedding triangles in other figures, or vice versa.
Here are just a few examples, which include triangles within and outside of squares, rectangles, and circles:
Today, we’ll talk about some crucial connections that are often made between triangles and other figures, starting with the 45-45-90 triangle, also known as the isosceles right triangle.
You’ve probably seen a rectangle split in two along one of its diagonals to produce two right triangles:
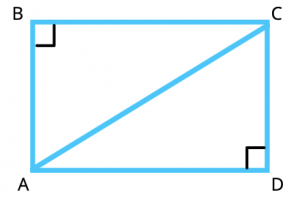
But one of the oft-overlooked basic geometric truths is that when that rectangle is a square (and yes, remember a square is a type of rectangle), the diagonal splits the square into two isosceles right triangles. This makes sense when you think about it, because the diagonal bisects two 90-degree angles to give you two 45-degree angles:
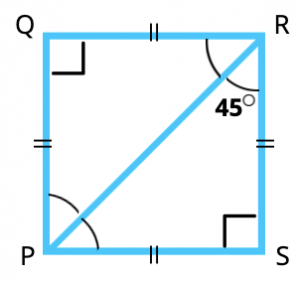
(For clarification, the diagonal of a rectangle is a bisector when the rectangle is a square, but it is not a bisector in any other case.)
Another very common combination of shapes in more difficult GMAT Geometry problems is triangles with circles. This can manifest itself in three common ways:
Triangles Created Using the Central Angle of a Circle
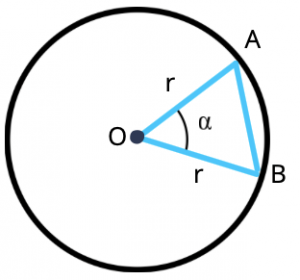
In this case, notice that two of the sides of the triangle are radii (remember, a radius is any line segment from the center of the circle to its circumference). What does that guarantee about the triangle?
Since two side are of equal length, the triangle is automatically isosceles. Remember that the two angles opposite those two sides are also of equal measure. So any triangle with the center of the circle as one vertex and points along the circumference as the other two vertices will automatically be an isosceles triangle.
Inscribed Triangles
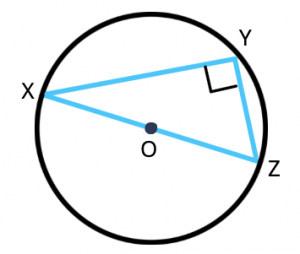
An inscribed triangle is any triangle with a circle’s diameter as one of its sides and a vertex along the circumference. And a key thing to note: an inscribed triangle will ALWAYS be a right triangle. So even if you don’t see the right angle marked, you can rest assured the inscribed angle at that third vertex is 90 degrees.
Squares and Rectangles Inscribed in Circles
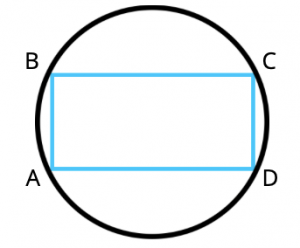
What’s important to note here is that the diagonal of the rectangle (or square) is equivalent to the diameter of the circle.
Now that we’ve seen a few common relationships between triangles and other figures, let’s take a look at an example Official Guide problem:
A small, rectangular park has a perimeter of 560 feet and a diagonal measurement of 200 feet. What is its area, in square feet?
A) 19,200
B) 19,600
C) 20,000
D) 20,400
E) 20,800
Explanation
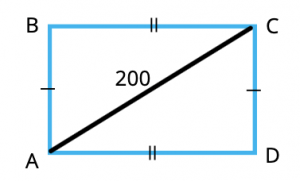
The diagonal splits the rectangular park into two similar triangles:
Use Signals to Avoid Algebra
It can be tempting to then jump straight into algebra. The formulas for perimeter and diagonal are P = 2L + 2W an D2 = L2 + W2, respectively, where L and W are the length and width of the rectangle. The second formula, you’ll notice, arises out of the Pythagorean Theorem, since we now have two right triangles. We are trying to find area, which is LW, so we could set out on a cumbersome algebraic journey.
However, let’s try to use some SIGNALS the problem gives us and our knowledge of how the GMAT operates to see if we can short-circuit this problem.
We know the GMAT is fond of both clean numerical solutions and common Pythagorean triples. The large numbers of 200 for the diagonal and 560 for the perimeter don’t change that we now have a very specific rectangle (and pair of triangles). Thus, we should suspect that one of our basic Pythagorean triples (3-4-5, 5-12-13, 7-24-25) is involved.
Could it be that our diagonal of 200 is the hypotenuse of a 3-4-5 triangle multiple? If so, the 200 would correspond to the 5, and the multiplying factor would be 40. That would also mean that the legs would be 3*40 and 4*40, or 120 and 160.
Does this check out? Well, we’re already told the perimeter is 560. Adding 160 and 120 gives us 280, which is one length and one width, or half the perimeter of the rectangle. We can then just double the 280 to get 560 and confirm that we do indeed have the correct numbers. The length and width of the park must be 120 and 160. No algebra necessary.
Now, to get the area, we just multiply 120 by 160 to get 19,200 and the final answer of A.
Check out the following links for our other articles on triangles and their properties:
A Short Meditation on Triangles
The 30-60-90 Right Triangle
The 45-45-90 Right Triangle
The Area of an Equilateral Triangle
Isosceles Triangles and Data Sufficiency
Similar Triangles
3-4-5 Right Triangle
5-12-13 and 7-24-25 Right Triangles
By: Rich Zwelling, Apex GMAT Instructor
Date: 16th February, 2021
