Triangles, and Geometry in general, are something you need to master as much as you can before your GMAT exam. It can seem complicated in the beginning, but once you start working with different geometric objects and its shapes, thing will definitely get easier. The topic of this article is similar triangles. One of the most important things to highlight here is that “similar” does not necessarily mean “identical.” Two triangles can be similar without being the same size. For example, take the following:
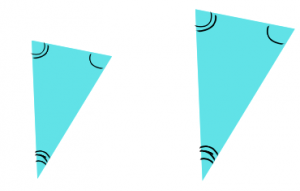
Even though the triangles are of different size, notice that the angles remain the same. This is what really defines the triangles as similar.
Now, what makes this interesting is that the measurements associated with the triangle increase proportionally. For example, if we were to present a triangle with lengths 3, 5, and 7, and we were to then tell you that a similar triangle existed that was twice as large, the corresponding side lengths of that similar triangle would have to be 6, 10, and 14. (This should be no surprise considering our lesson on multiples of Pythagorean triples, such as 3-4-5 leading to 6-8-10, 9-12-15, etc.)
You can also extend this to Perimeter, as perimeter is another one-dimensional measurement. So, if for example we ask:
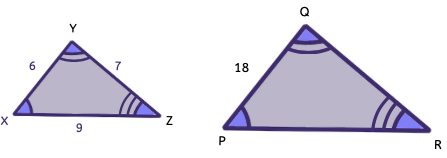
A triangle has line segments XY = 6, YZ = 7, and XZ = 9. If Triangle PQR is similar to Triangle XYZ, and PQ = 18, as shown, then what is the perimeter of Triangle PQR?
Answer: Perimeter is a one-dimensional measurement, just as line segments are. As such, since PQ is three times the length of XY, that means the perimeter of Triangle PQR will be three times the perimeter of Triangle XYZ as well. The perimeter of Triangle XYZ is 6+7+9 = 22. We simply multiply that by 3 to get the perimeter of Triangle PQR, which is 66.
Things can get a little more difficult with area, however, as area is a two-dimensional measurement. If I double the length of each side of a triangle, for example, how does this affect the area? Think about it before reading on…
Scenario
Suppose we had a triangle that had a base of 20 and a height of 10:
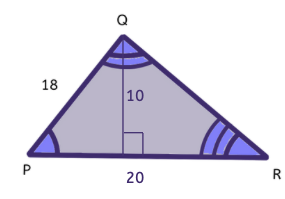
The area would be 20*10 / 2 = 100.
Now, if we double each side of the triangle, what effect does that have on the height? Well, the height is still a one-dimensional measurement (i.e. a line segment), so it also doubles. So the new triangle would have a base of 40 and a height of 20. That would make the area 40*20 / 2 = 400.
Notice that since the original area was 100 and the new area is 400, the area actually quadrupled, even though each side doubled. If the base and height are each multiplied by 2, the area is multiplied by 22. (There’s a connection here to units, since units of area are in square measurements, such as square inches, square meters, or square feet.)
Now, let’s take a look at the following original problem:
Triangle ABC and Triangle DEF are two triangular pens enclosing two separate terrariums. Triangle ABC has side lengths 7 inches, 8 inches, and 10 inches. A beetle is placed along the outer edge of the other terrarium at point D and traverses the entire perimeter once without retracing its path. When finished, it was discovered that the beetle took three times as long as it did traversing the first terrarium traveling at the same average speed in the same manner. What is the total distance, in inches, that the beetle covered between the two terrariums?
A) 25
B) 50
C) 75
D) 100
E) 125
Explanation
This one has a few traps in store. Hopefully you figured out the significance of the beetle taking three times as long to traverse the second terrarium at the same average speed: it’s confirmation that the second terrarium has three times the perimeter of the first. At that point, you can deduce that, since the first terrarium has perimeter 7+8+10 = 25, the second one must have perimeter 25*3 = 75. However, it can be tempting to then choose C, if you don’t read the question closely. Notice the question effectively asks for the perimeters of BOTH terrariums. The correct answer is D.
GMAT Triangle Series Articles
A Short Meditation on Triangles
The 30-60-90 Right Triangle
The 45-45-90 Right Triangle
The Area of an Equilateral Triangle
Triangles with Other Shapes
Isosceles Triangles and Data Sufficiency
Similar Triangles
3-4-5 Right Triangle
5-12-13 and 7-24-25 Right Triangles
