By: Rich Zwelling, Apex GMAT Instructor
Date: 12th January, 2021
The 5-12-13 and 7-24-25 Right Triangles
Although the 3-4-5 right triangle is by far the most common of the so-called “Pythagorean triples” tested on the GMAT, there are a few others worth knowing. First, a little review:
You’ll recall that the Pythagorean Theorem (a² + b² = c²) holds for any right triangle where a and b are the two legs and c is the hypotenuse, and that the 3-4-5 triangle represents the smallest such triangle with all integer side lengths:
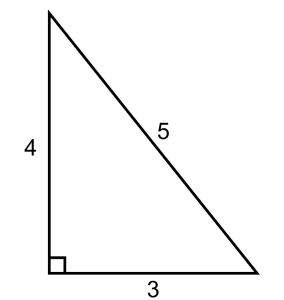
This works not only for 3-4-5 but also for 6-8-10, 9-12-15, or any other multiples of each side length.
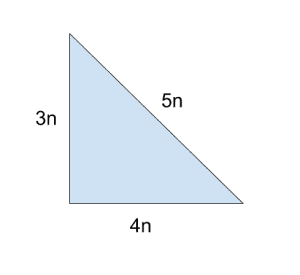 No matter what positive integer n you choose for the figure above, you will produce a valid right triangle.
No matter what positive integer n you choose for the figure above, you will produce a valid right triangle.
So now we come to the main topic: what are some other common “Pythagorean triples” the GMAT may test? The next base triples that fit the Pythagorean Theorem are 5-12-13 and 7-24-25. These work because if you check the arithmetic, 5² + 12² = 13² and 7² + 24² = 25²:
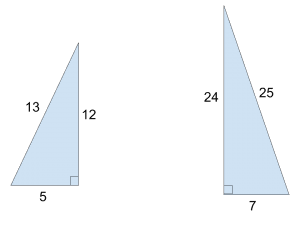
As we’ve continually discussed, however, your success on more difficult GMAT problems will require you to go beyond mere rote memorization. Let’s take a look at an Official Guide Data Sufficiency problem that illustrates how the test can force you to engage some higher-level reasoning skills:
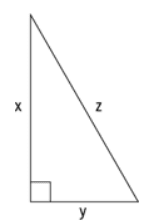
If A is the area of a triangle with sides of lengths x, y, and z as shown above, what is the value of A?
(1) z = 13
(2) A = 5y/2
Give it a try on your own before reading any further.
As with any Data Sufficiency question, let’s identify what we’re asked to find. A represents the area of the triangle, which is found by multiplying base by height and dividing by 2. That means A = xy/2, since x and y represent the height and base, respectively.
Remember, it helps to frame Data Sufficiency questions in terms of what information you need to get to the answer. We need to know the individual values of x and y. Or, as a matter of fact, we could have sufficiency if we knew xy as a product, even if we didn’t know the values of x and y, individually. For example, on a different problem with the same question, if the test had said that the product of the base and height were 30, that would have been sufficient, as that would be enough for us to deduce that the area is 15.
You can save yourself much time and mental energy by having a solid idea of what information you need from the statements for sufficiency before you actually view the statements.
Now that we know what information we need for sufficiency, let’s examine each statement on its own. Statement (1) should get you thinking about the 5-12-13 right triangle, as it tells us that the hypotenuse is 13. But be careful: this is where rote memorization only goes so far (and may actually get in the way).
Does knowing that the hypotenuse is 13 guarantee that the other sides are 5 and 12? For all we know, they could be non-integers that fit a² + b² = 13². In fact, a and b could be equal — remember that we can’t assume that the figures are drawn to scale. Without a clear idea of what the base and height are, we cannot get a consistent product for xy. Statement (1) is INSUFFICIENT on its own.
Statement (2) is more complicated, as we have two variables, one of which is the area. But we already discussed that A = xy/2, so we can do a substitution:
A = 5y/2
xy/2 = 5y/2
At this point, we can see that the sides are identical, except that the x on the left has been replaced by a 5 on the right. Therefore, x must be 5. Again, this should get us thinking about the 5-12-13 triangle. But we should again remember that this alone does not guarantee that the other sides are 12 and 13. Even though x is 5, there could be multiple values for y, and that means multiple values for the product xy. Statement (2) is also INSUFFICIENT on its own.
This narrows the answer choices down to C (statements sufficient together) and E (statements insufficient together).
This is where previous knowledge of the 5-12-13 triangle helps. Ideally, once you see that the statements together tell you that x=5 and z=13, you will know without much thought that y must be 12. You won’t bother using the Pythagorean theorem and you certainly won’t wonder if y could have multiple values.
Without knowledge of the 5-12-13, one trap a test-taker could possibly fall into is viewing the two statements and noticing that there are 3 variables and only 2 equations. We need a full 3 equations with 3 variables if we’re going to solve for all 3 variables, and that may lead some to prematurely conclude that the answer is E.
However, why is that a false conclusion?
Well, we’re not trying to solve for all variables. We’re only solving for one. It’s possible to solve for one variable, even if there are fewer equations than variables.
In this case, now that we know that x=5 and y=12, we have our base and height, and we can solve for A, the area of the triangle. Note that I’m not going to bother solving, because for sufficiency, I don’t need to. I only care that I CAN solve. The final answer is (C).
We’ve now talked about the various Pythagorean triples and special right triangles. Next time, we’ll talk about how triangles can appear within OTHER shapes. In the meantime, solve some problem practice problems, keep track of your progress, as well as consider working with a GMAT tutor should you feel like you need somebody professional in the field to help you succeed during your GMAT prep. And to tide yourself over, you can also link to our other article about triangles:
A Short Meditation on Triangles
The 30-60-90 Right Triangle
The 45-45-90 Right Triangle
The Area of an Equilateral Triangle
Triangles with Other Shapes
Isosceles Triangles and Data Sufficiency
Similar Triangles
3-4-5 Right Triangle
5-12-13 and 7-24-25 Right Triangles
