As you will definitively have to deal with radicals on the GMAT and Executive Assessment (EA), we’ve put together an article for you to master the topic. Addition is “undone” by subtraction, multiplication is “undone” by division, and the powers notated by exponents are “undone” with a piece of notation called a radical.
√ the radical
The number, variable, or expression inside or under the radical is referred to as the radicand, and sometimes there is a small number called the index nestled in the “crook” of the radical.
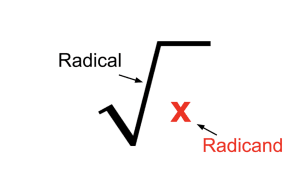
When no index appears, the index is understood to have a value of 2. (This will make sense momentarily.) Unfortunately there exists no one-word name – like “addition” or “multiplication” for what exponents do. For an exponent of n, we use the phrase “raising to the nth power.” For a radical with an index of n, we use the phrase “taking the nᵗʰ root.” Exponents notate powers, radicals notate roots. If there is an “invisible” index of 2, the notated value is called the square root of the radicand (the number inside or under the radical). If the index is 3, the notated value is called the cube root of the radicand. For all integers above 3, we use the usual ordinals (fourth root, fifth root, etc.)
√36 the square root of 36
³√125 the cube root of 125
⁴√81 the fourth root of 81
If you’ve studied your powers chart from the first article in this series, you should recognize those radicands. Here’s a simple way to represent how radicals “undo” exponents:
xⁿ = a ⁿ√a = x
If the nᵗʰ power of x equals a, then the nth root of a equals x. Radical expressions like this notate the number x which, when multiplied by itself n times (or, to use exponents, raised to the nᵗʰ power), equals a. Exponential expressions “start with” a base and raise it to a power, notating a value (in our case the variable a). Radical expressions “start with” the full value notated by some exponential expression and use the exponent from this expression as a root, notating the base of the exponential expression (in our case the variable x). To use our examples above:
√36 the square root of 36 x² = 36, x = 6
³√125 the cube root of 125 x³ = 125, x = 5
⁴√81 the fourth root of 81 x⁴ = 81, x = 3
Now you have another reason to learn your common powers: when you see one of those special numbers underneath a radical, you can quickly evaluate the radical expression. This is akin to how knowing your times tables makes division really easy.
Another quality of roots is that they can be “translated” into fractional exponents according to the following rule:
ⁿ√(xᵃ) = xᵃ⁄ⁿ
(ⁿ√x)ᵃ = xᵃ⁄ⁿ
Two forms are shown because the position of the exponent a is irrelevant. It can be considered “within” and “before” the radical operation as in the first version or “above” and “after” the radical operation as in the second version.
As always, this rule can be reversed.
xᵃ⁄ⁿ = n√(xa)
xᵃ⁄ⁿ = (n√x)a
Seeing fractional exponents written in GMAT and EA questions is quite rare, but sometimes a given radical expression that doesn’t break down easily is better notated as a fractional exponent for the sake of seeing potential algebraic simplifications.
This correlation between radicals and fractional exponents brings up the key point that radicals and exponents are just different – or reverse – ways of notating the same thing. Therefore all the exponent rules apply to radicals as well. The radical versions appear less frequently than the exponent versions but are still valuable pieces in your toolkit.
Equal bases with different roots are rather unusual; combining or collapsing roots with the same index is certainly more common. And just as we can have a “power of a power,” we can have a “root of a root.”
These rules bring up a final important point about radical expressions: they often need to be simplified. Mathematicians don’t like to leave numbers inside radicals when it is possible to express the same value differently, so many answer choices on the GMAT and EA involve simplified versions of radical expressions. Here’s an example for our purposes:
√630
We’ll have to get into some number properties to show what happens here. One of our radicals rules states that this radicand 630 can be broken into factors and represented as multiple radical expressions. Let’s break down 630 to its prime factors.
630 = 2 * 3² * 5 * 7
√630 = √(2 * 3² * 5 * 7)
Given an index of n, any n (number) of the same prime factor can be placed inside their own radical. Since we are taking a square root here (with an understood index of 2), we only need two of the same prime factor in order to do this. Here we can do it with our 3s.
√630 = √(2 * 3² * 5 * 7)
√630 = √(3²) * √(2 * 5 * 7)
Now the power of 2 and the root of 2 on the 3 cancel each other out.
√630 = √(32) * √(2 * 5 * 7)
√630 = 3 * √(2 * 5 * 7)
√630 = 3√70
When simplifying a radical expression, use the index as a key. Given an index of n, look for sets of n (number) of the same prime factor. For each of these sets, bring one of that prime factor out in front of the radical. Here’s another example:
³√8640
A key step to shortening the process is recognizing 216 as 63. Let’s line up our prime factors:
2⁶ * 3³ * 5
Remember that we’re taking a cube root this time, so we’re looking for sets of three of the same prime factor. We can make two sets from our 2s (since we have six of them) and one set from our 3s. So two 2s and one 3 come out from the radical, leaving only the 5.
³√8640 = 2 * 2 * 3 * ³√5 = 12³√5
To close this article, let’s try a couple of official GMAT problems involving radicals:
If n = √(16/81) , What is the value of √n?
(A) 1/9
(B) 1/4
(C) 4/9
(D) 2/3
(E) 9/2
This problem benefits from knowing both your rules and your powers. Knowing your rules lets you represent this radical expression as √16/√81, and knowing your powers lets you easily recognize √16 as 4 and √81 as 9, leading to answer choice C, 4/9. Here’s one more:
In the formula w= P/√v, Integers p and t are positive constants. If w=2 when v=1 and if w=1/2 when v=64, then t=
(A) 1
(B) 2
(C) 3
(D) 4
(E) 16
First we are told that w = 2 when v = 1.
2 = P / ᵗ√1
Here you need to recognize a fact we haven’t mentioned yet: that any root (or power) of 1 is still 1. This makes sense when you think about multiplying 1 by itself again and again – the value never changes. Therefore the denominator of the expression equals 1, and P must equal 2. Armed with this knowledge, you can make use of the second given fact: w = ½ when v = 64.
½ = 2/ᵗ√64
4 = ᵗ√64
If you recognize 64 as 26, you can use your “power to a power” rule to change 26 to 43, leading you to answer choice C.
This concludes our survey of radicals on the GMAT and EA. Next time we will introduce negatives to exponential expressions.
Contributor: Elijah Mize (Apex GMAT Instructor)
