Large Exponents and The Units Digit

Welcome to the final article in our series on exponents. Some GMAT and Executive Assessment (EA) quant problems feature impossibly large numbers like 2874⁵⁹. These are problems that you simply can’t hope to solve without a key piece of knowledge that we call units digit cycles.

Units digit cycles are extrapolations of the fact that the units digit of a product is entirely determined by the units digits of its factors. Consider the multiplication of two five-digit numbers ending with 3 and 7, respectively:

   _ _ _ _ 3

x _ _ _ _ 7

Since 3 * 7 = 21, the units digit of the product of these two numbers is 1. Here’s another example:

_ _ _ 6

x _ _ 4

Since 6 * 4 = 24, the units digit of the product of these two numbers is 4.

Now let’s look back at the powers chart from the very first article in this series:

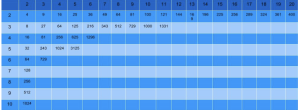
Hopefully, this chart is much more familiar to you now than it was when you read that first article. This time we’ve bolded the units digit of each value. Look down the 2 column, and you’ll see that the units digits follow the repeating cycle {2,4,8,6}. If we filled in a few more powers of 3, we could observe the repeating cycle {3,9,7,1}. For 5 and 6, the units digit never changes!

These cycles occur for powers of numbers because eventually, the units digit of a power of a given number will be the same as the units digit of the number itself. Let’s consider an example with the base 4. The units digit of 42 is determined by 4 * 4, which = 16. The units digit of 43 is determined by 6 * 4. 

 16

x 4

 64

Since 6 * 4 = 24, the units digit of 43 is 4. Now the units digit of 44 is determined by 4 * 4, which is where we began when evaluating 42. Thus, the units digits of powers of 4 will simply alternate between 4 and 6. Since the series of powers of n is formed by successive multiplications by n, then whenever nk has units digit d, the units digit of nk + 1 is the units digit of d * n.

Here’s a full list of units digit cycles for the digits 0 through 9:

0: {0}

1: {1}

2: {2,4,8,6}

3: {3,9,7,1}

4: {4,6}

5: {5}

6: {6}

7: {7,9,1,3}

8: {8,4,2,6}

9: {9.1}

These cycles are a key piece of memorization for GMAT/EA quant. Let’s apply them to an official problem:

If n = 33⁴³ + 43³³, what is the units digit of n?

(A) 0

(B) 2

(C) 4

(D) 6

(E) 8

We’ll need to find and then add the units digits of 33⁴³ and 43³³. Remember that only the units digit of the base matters. The units digit of 33⁴³ is the same as the units digit of 3⁴³. And the units digit of 43³³ is the same as the units digit of 3³³.

The units digit of 343 is the units digit of the 43rd member of the cycle from above: {3,9,7,1}. The cycle repeats like this:

3 9 7 1 3 9 7 1 3 9 7 1 3 9 7 1 . . .

We don’t want to keep writing this all the way to the 43rd member, but we can use division as a shortcut. Divide the exponent by the number of members of the cycle for the units digit of the base, and the remainder of this division tells you which member of the cycle to take. The quotient of this division corresponds to the number of times you would count all the way through the cycle, which is irrelevant. The remainder tells you where in the cycle you “end up” when you reach the member you’re looking for.

In this case, our exponent is 43, and the units digit of our base is 3, which has a cycle with 4 members. So we need to divide 43 / 4 and take the remainder. Since the remainder of 43 / 4 is 3, the units digit of 3⁴³ (and of 3343) is the third member of the cycle {3,9,7,1}, which is 7.

Let’s run this again on the other value in the problem: 43³³. Again the units digit of the base is 3, so we will use the cycle {3,9,7,1}. We still have four members in the cycle, so the remainder of the exponent divided by 4 tells us which member of the cycle to take. This time the exponent is 33. The remainder of 33/4 is 1, so we need the first member of the cycle {3,9,7,1}, which is 3.

As a final step in finding the units digit of 33⁴³ + 43³³, we need to add the units digits of 3343 and 4333, which we have found to be 7 and 3. Since 7 + 3 = 10, the units digit of 33⁴³ + 43³³ = 0. So the correct answer is A.

Some units digits problems ask about remainders. Here’s an example and the last official problem in our exponents series:

What is the remainder when 3²⁴ is divided by 5?

(A) 0

(B) 1

(C) 2

(D) 3

(E) 4

When you see a very large exponent like 24, finding the units digit of the exponential expression is almost always required. We are working with a base of 3 again, so the units digit cycle is {3,9,7,1}. The exponent, 24, is a multiple of the number of members (4) of this cycle, so the remainder 24 / 4 is 0 and the units digit of 3²⁴ is the last member of the cycle: 1. So the number 3²⁴ has form _ _ _ _ _ 1. There are certainly more than 6 digits in 3²⁴, but all that matters here is the units digit. All multiples of 5 have units digits of 5 or 0, and a number with a units digit of 1 (like 3²⁴) is 1 greater than some number with a units digit of 0. Therefore the remainder of 3²⁴ / 5 is 1, and the correct answer is B.

This concludes our article on large exponents and the units digit, and our series of articles covering exponents on the GMAT and EA. If you missed any of the previous articles (there are 10 in total), search them out and read them through to strengthen your GMAT/EA quant readiness.

Are there any questions that you still have about the exponents on the GMAT/EA? Register now for a free 30-minute free consultation with one of our top tutors.

Contributor: Elijah Mize (Apex GMAT Instructor)